THD. Short for total harmonic distortion, its measurements show how much harmonic artifacts hifi components inject. It's a complex subject with a math aspect. As an Irish country bumpkin, I give you the super-lite version. First we need to understand what harmonics are. If you've ever overblown an empty bottle or spun a whirly tube fast enough, you've met at least the 2nd harmonic. It occurred an octave above the bottle's or tube's resonant frequency. If you blew even harder or spun the tube faster still, you got to the 3rd harmonic which occurs a perfect fifth above the 2nd. Octave, fifth, third etc describe frequency ratios or spacing against the ground or fundamental tone. An octave occurs at exactly twice the fundamental frequency for example.
For our purposes it suffices to know that unlike a synthesizer generating a test tone, acoustic instruments and voices don't produce pure sinewaves. As the word 'harmonics' suggests, overtones play complex harmonies above the fundamental. We don't usually perceive harmonics as separate tones because they're very low in amplitude. They're embedded or mixed in completely and create timbre. Timbre is the harmonic signature of an instrument or singer. It's what gives a voice or player their unique tone. It's how we distinguish five different voices all of which sing the exact same fundamental. The overtone content—the distribution of harmonics and their relative prominence—creates different tone colours. When somebody is hoarse or deliberately strains their vocal cords, we often make out one or two harmonics as simultaneously occurring separate tones. Mongolian throat singers are trained to amplify harmonics so that we can hear them as actual chords.
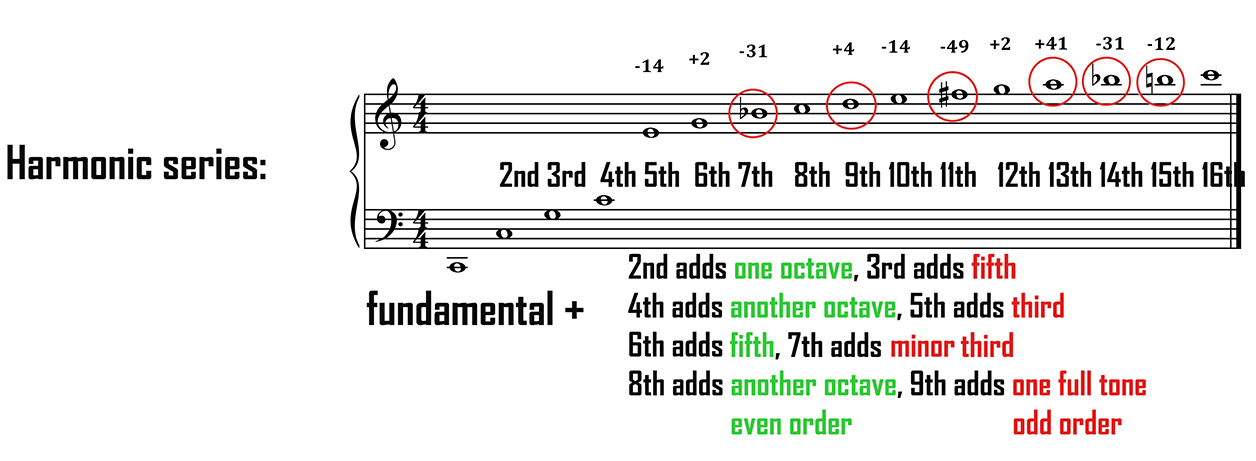To really make the point of the dissonant notes circled red, imagine hitting a piano's white key then the black key right next to it, then the next white key and black key – all at once. Voilà. Now seriously reduce the amplitude of anything but the first key and you've got the higher-order THD coloration. It's really not pretty.
The easiest way to explain the effect harmonics have on tone colour is to take a C-major chord in the even-tempered Western scale and call out those overtones which don't belong. The 2nd, 4th, 6th, 8th, 10th, 12th and 16th harmonic all belong because they space at even octaves, thirds or fifths to become 'C', 'E' or 'G', the three tones which make up a C-major chord. The 3rd and 5th harmonic belong too because they too occur at a fifth and third so again 'G' and 'E'. An octave is perfectly neutral. Adding a fifth creates a certain freshness. The third determines whether we're in C major or minor. If it's 'E' we're in major. If 'E' steps down half a tone, we're in C minor. That makes the third less neutral and more determinant. It's the most common choice for a backup singer's harmony behind the lead vocal. The 7th harmonic becomes B-flat which in Jazz is a 'blue' note. It injects deliberate sharpness. The 9th harmonic becomes a 'D' which sits a full tone apart from a 'C' so rubs. The 11th harmonic becomes an F-sharp which is a half tone beneath 'G' to really rub. Rubbing means minor or major dissonance. Now we appreciate why low-order even harmonics have an enriching effect. Except for the 6th, it's pure octave doubling, tripling and quadrupling. That's comparable to having a child, woman and man sing the same note in unison. At their natural pitch, the man will sing an octave below the woman, the child an octave above her. The same tone in triplicate spread across three octaves sounds a lot richer than any singer by themselves. We also appreciate how the low-order odd harmonics of the 3rd and 5th are just as benign but create minor keenness which octaves don't.
It's the higher odd-order harmonics of the 7th and up which add dissonance to be problematic even in minute doses. And so far we've only considered what happens if we play single notes at a time. Once we add stacked harmonies and melodies which cross them chromatically, things get rapidly more complex. The takeaway is that the 2nd, 4th and 8th harmonic are benign no matter what since higher octaves can only add richness, never any dissonance or cool/crisp colouration. It's why low even-order THD has a pleasing filler effect even at high SET doses whilst just a homeopathic dose of the 7th, 9th or 11th really doesn't belong even on simple girl 'n' guitar fare and becomes outright offensive on harmonically complex dense music. Low odd-order THD of the 3rd and 5th injects freshness or some 'neon' to counter the richer creamer 'pastel' effect of the 2nd and 4th. But the even 14th really goes blue so calling odd-order THD bad and all even-order good is too simplistic. What's correct to say is that odd-order THD breaks bad a lot sooner. Equally correct is that low-order THD even or odd has 50:50 admirers according to the sales figures of Pass Labs and FirstWatt which produce electronics of either flavour. I used to be a 2nd-harmonic guy. Today I'm a 3rd-harmonic disciple. Tastes can change and virtually all gain circuits have some THD. We must pick our poison.
A 2nd-harmonic SET, 3rd-harmonic class AB transistor amp plus ultra-high feedback class D amp. Different THD, different sonic profiles.
Those who look at THD for just the lowest figures without considering which harmonics are involved overlook an important colorant. Copious negative feedback can cancel a lot of THD yet the high gain required to apply a lot of NFB could require extra gain stages which upshift the THD spectrum into the higher ranges. Whilst overall THD could read very low indeed, a close look at the remnants could show very high harmonics far past the 9th into higher double digits. Meanwhile a simple classic SET may have high 2nd/4th-harmonic distortion but nothing above it. Now you appreciate why the SET will sound a lot richer whilst our super-low THD amp could have a certain bite, metallic rust or flinch factor that becomes annoying. Making sense of THD thus doesn't just look at a solitary number on a spec sheet. It looks at the distribution of said THD. And even that's simplistic because that distortion changes with load. Playing louder tends to increase distortion. What may not factor at background levels could well bare its teeth at high SPL. Does THD now rise linear or do certain areas of the overall distortion envelope gain more prominence? If so, which? Argh, now we're breaching more advanced areas where this country bumpkin falls off his super-lite turnip truck. Feckin' eejit!
